已知兩個不同點畫七個圓，得到另兩個正方形頂點

ejsoon

現在有不同的兩個點，只給你一個圓規，畫七個圓，得到另兩個點，這兩個點跟開始的兩個點是在同一平面的一個正方形的四個頂點。

（此題寡人暫時沒有解出来）

lsr314

【震惊】只用圆规就能构造正方形，99%的人都不知道！

风云剑

这题是不是还有个限制，给定的两点是正方形相邻的两顶点？
否则一个中垂线不就行了

ejsoon

风云剑 发表于 2021-9-29 17:26
这题是不是还有个限制，给定的两点是正方形相邻的两顶点？
否则一个中垂线不就行了

是的，不過即便是對角點，好像也不是很好做。因為只能用圓規，不能用尺。

ejsoon

雖然答案近在咫尺，但是寡人希望自己找到答案，然而時過多日依然沒有解開…

mathe

步数不多，计算机求解是很容易的。
我们处理对象有点、圆、长度
其中除了给定的两个初始点(0,0),(1,0),其余的点都表示为两个圆的交点。而长度都表示为两个点的距离，圆由一个点和一个长度决定。
有点麻烦的是过一个点可以超过两个圆，同样代表同一个长度的可以有多个点对，通常我们可以随机选择一个最早遇到的匹配项就差不多是最优的了。

我们反复利用上面模式进行迭代:点->长度->圆->点->长度->圆，..直到产生目标长度即可($\sqrt{2}$), 最后在根据生成关系，反向寻找做图关系即可。

mathe

Require init Point[0]: (0.000000,0.000000)
Require init Point[1]: (1.000000,0.000000)
Require Distance[1] value 1.000000 from Point[0] to Point[1]
Require Circle[1] from Point[0] Radius 1.000000 from Distance[1]
Require Circle[3] from Point[1] Radius 1.000000 from Distance[1]
Require Point[2]: (0.500000,0.866025) from Circle [1] [3]
Require Point[3]: (0.500000,-0.866025) from Circle [1] [3]
Require Distance[2] value 1.732051 from Point[2] to Point[3]
Require Circle[6] from Point[2] Radius 1.732051 from Distance[2]
Require Circle[7] from Point[3] Radius 1.732051 from Distance[2]
Require Circle[9] from Point[2] Radius 1.000000 from Distance[1]
Require Circle[11] from Point[3] Radius 1.000000 from Distance[1]
Require Point[40]: (1.192219,0.144338) from Circle [9] [7]
Require Point[47]: (-0.192219,-0.144338) from Circle [11] [6]
Found distance 1.414214 from Point[40] to Point[47]

ejsoon

最終寡人是通過這個網頁的介紹得到答案的，同時寡人認為，如果要寡人自己尋找到解答，那還是太難了。

（如果用其它地方介紹的方法）即便拿到7L7E，剩下的3V也不是很好拿。

ejsoon

不過根據知乎，以及2#的視頻，這道題至少有三種解法。

那麼，如何證明最優解法是畫七個圓？

mathe

前面一个公式里面b1输入成b2,找了好久才找出bug.
现在程序可以找出好多解，代码会输出需要做出的圆，都是做出6个圆后得到点(0,1)，所以最后再添加一个圆就可以得到(1,1)。

Found one Solution by intersect circle[4](0.500000,0.866025:1.000000) & circle[600](0.728714,0.684819:0.736595):
Circle[0]: Center(0.000000,0.000000), Radius 1.000000
Circle[1]: Center(1.000000,0.000000), Radius 1.000000
Circle[2]: Center(0.000000,0.000000), Radius 1.732051
Circle[4]: Center(0.500000,0.866025), Radius 1.000000
Circle[137]: Center(1.500000,-0.866025), Radius 1.732051
Circle[600]: Center(0.728714,0.684819), Radius 0.736595


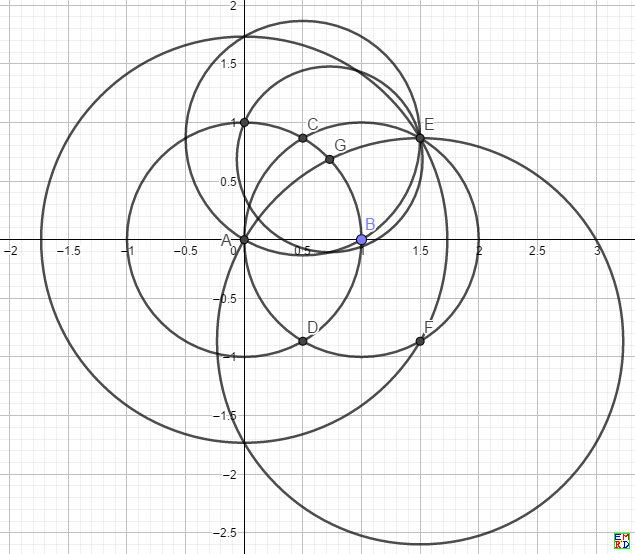
Found one Solution by intersect circle[4](0.500000,0.866025:1.000000) & circle[600](0.728714,0.684819:0.736595):
Circle[0]: Center(0.000000,0.000000), Radius 1.000000
Circle[1]: Center(1.000000,0.000000), Radius 1.000000
Circle[4]: Center(0.500000,0.866025), Radius 1.000000
Circle[6]: Center(0.500000,-0.866025), Radius 1.000000
Circle[137]: Center(1.500000,-0.866025), Radius 1.732051
Circle[600]: Center(0.728714,0.684819), Radius 0.736595


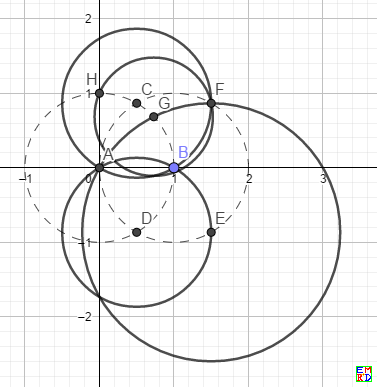
Found one Solution by intersect circle[4](0.500000,0.866025:1.000000) & circle[686](0.271286,0.684819:1.000000):
Circle[0]: Center(0.000000,0.000000), Radius 1.000000
Circle[1]: Center(1.000000,0.000000), Radius 1.000000
Circle[3]: Center(1.000000,0.000000), Radius 1.732051
Circle[4]: Center(0.500000,0.866025), Radius 1.000000
Circle[86]: Center(-0.500000,-0.866025), Radius 1.732051
Circle[686]: Center(0.271286,0.684819), Radius 1.000000

Found one Solution by intersect circle[4](0.500000,0.866025:1.000000) & circle[686](0.271286,0.684819:1.000000):
Circle[0]: Center(0.000000,0.000000), Radius 1.000000
Circle[1]: Center(1.000000,0.000000), Radius 1.000000
Circle[4]: Center(0.500000,0.866025), Radius 1.000000
Circle[6]: Center(0.500000,-0.866025), Radius 1.000000
Circle[86]: Center(-0.500000,-0.866025), Radius 1.732051
Circle[686]: Center(0.271286,0.684819), Radius 1.000000

Found one Solution by intersect circle[6](0.500000,-0.866025:1.000000) & circle[612](0.728714,-0.684819:0.736595):
Circle[0]: Center(0.000000,0.000000), Radius 1.000000
Circle[1]: Center(1.000000,0.000000), Radius 1.000000
Circle[2]: Center(0.000000,0.000000), Radius 1.732051
Circle[6]: Center(0.500000,-0.866025), Radius 1.000000
Circle[120]: Center(1.500000,0.866025), Radius 1.732051
Circle[612]: Center(0.728714,-0.684819), Radius 0.736595

Found one Solution by intersect circle[6](0.500000,-0.866025:1.000000) & circle[612](0.728714,-0.684819:0.736595):
Circle[0]: Center(0.000000,0.000000), Radius 1.000000
Circle[1]: Center(1.000000,0.000000), Radius 1.000000
Circle[4]: Center(0.500000,0.866025), Radius 1.000000
Circle[6]: Center(0.500000,-0.866025), Radius 1.000000
Circle[120]: Center(1.500000,0.866025), Radius 1.732051
Circle[612]: Center(0.728714,-0.684819), Radius 0.736595

Found one Solution by intersect circle[6](0.500000,-0.866025:1.000000) & circle[698](0.271286,-0.684819:1.000000):
Circle[0]: Center(0.000000,0.000000), Radius 1.000000
Circle[1]: Center(1.000000,0.000000), Radius 1.000000
Circle[3]: Center(1.000000,0.000000), Radius 1.732051
Circle[6]: Center(0.500000,-0.866025), Radius 1.000000
Circle[69]: Center(-0.500000,0.866025), Radius 1.732051
Circle[698]: Center(0.271286,-0.684819), Radius 1.000000

Found one Solution by intersect circle[6](0.500000,-0.866025:1.000000) & circle[698](0.271286,-0.684819:1.000000):
Circle[0]: Center(0.000000,0.000000), Radius 1.000000
Circle[1]: Center(1.000000,0.000000), Radius 1.000000
Circle[4]: Center(0.500000,0.866025), Radius 1.000000
Circle[6]: Center(0.500000,-0.866025), Radius 1.000000
Circle[69]: Center(-0.500000,0.866025), Radius 1.732051
Circle[698]: Center(0.271286,-0.684819), Radius 1.000000

Found one Solution by intersect circle[69](-0.500000,0.866025:1.732051) & circle[1190](-0.271286,0.684819:1.444003):
Circle[0]: Center(0.000000,0.000000), Radius 1.000000
Circle[1]: Center(1.000000,0.000000), Radius 1.000000
Circle[7]: Center(0.500000,-0.866025), Radius 1.732051
Circle[69]: Center(-0.500000,0.866025), Radius 1.732051
Circle[102]: Center(-1.000000,0.000000), Radius 1.000000
Circle[1190]: Center(-0.271286,0.684819), Radius 1.444003

Found one Solution by intersect circle[86](-0.500000,-0.866025:1.732051) & circle[996](-0.271286,-0.684819:1.444003):
Circle[0]: Center(0.000000,0.000000), Radius 1.000000
Circle[1]: Center(1.000000,0.000000), Radius 1.000000
Circle[5]: Center(0.500000,0.866025), Radius 1.732051
Circle[86]: Center(-0.500000,-0.866025), Radius 1.732051
Circle[102]: Center(-1.000000,0.000000), Radius 1.000000
Circle[996]: Center(-0.271286,-0.684819), Radius 1.444003

Found one Solution by intersect circle[104](-1.000000,0.000000:2.000000) & circle[293](-0.686141,0.396143:1.732051):
Circle[0]: Center(0.000000,0.000000), Radius 1.000000
Circle[1]: Center(1.000000,0.000000), Radius 1.000000
Circle[3]: Center(1.000000,0.000000), Radius 1.732051
Circle[7]: Center(0.500000,-0.866025), Radius 1.732051
Circle[104]: Center(-1.000000,0.000000), Radius 2.000000
Circle[293]: Center(-0.686141,0.396143), Radius 1.732051

Found one Solution by intersect circle[119](1.500000,0.866025:1.000000) & circle[1228](1.271286,0.684819:0.736595):
Circle[0]: Center(0.000000,0.000000), Radius 1.000000
Circle[1]: Center(1.000000,0.000000), Radius 1.000000
Circle[7]: Center(0.500000,-0.866025), Radius 1.732051
Circle[119]: Center(1.500000,0.866025), Radius 1.000000
Circle[153]: Center(2.000000,0.000000), Radius 1.000000
Circle[1228]: Center(1.271286,0.684819), Radius 0.736595

Found one Solution by intersect circle[136](1.500000,-0.866025:1.000000) & circle[1034](1.271286,-0.684819:0.736595):
Circle[0]: Center(0.000000,0.000000), Radius 1.000000
Circle[1]: Center(1.000000,0.000000), Radius 1.000000
Circle[5]: Center(0.500000,0.866025), Radius 1.732051
Circle[136]: Center(1.500000,-0.866025), Radius 1.000000
Circle[153]: Center(2.000000,0.000000), Radius 1.000000
Circle[1034]: Center(1.271286,-0.684819), Radius 0.736595